AP Statistics Course Outline

• Ap Statistics
• Ap Statistics Formula Sheet

I. Exploring Data: Describing patterns and departures from patterns (20%–30%) Exploratory analysis of data makes use of graphical and numerical techniques tostudy patterns and departures from patterns. Emphasis should be placed oninterpreting information from graphical and numerical displays and summaries.

A.Constructing and interpreting graphical displays of distributions of univariate data (dotplot, stemplot, histogram, cumulative frequency plot)

The AP Statistics Exam is made up of two parts: a multiple-choice section and a free response section. The entire test lasts three hours. Here's how the time is allotted. AP Statistics Formula Sheet and Tables (.pdf/1 MB) Recommended for students typing responses. Microsoft Word, Google Docs, Notes, or another app to enter and save text; Keyboarding Tip Sheet for 2020 AP Statistics Exams (.pdf/472KB) Permitted. Your class notes or study guides; Textbooks and other classroom resources; Previous assignments. Spencer's Statistics 2020-2021. Home AP Statistics Regular Statistics About Me Click on the Button. Download and Save. 2021 Student Notes: Blank. AP Statistics Course and Exam Description This is the core document for the course. It clearly lays out the course content and describes the exam and the AP Program in general.

1. Center and spread

2. Clusters and gaps

3. Outliers and other unusual features

4. Shape

B. Summarizing distributions of univariate data

1. Measuring center: median, mean

2. Measuring spread: range, interquartile range, standard deviation

3. Measuring position: quartiles, percentiles, standardized scores(z-scores)

4. Using boxplots

5. The effect of changing units on summary measures

C. Comparing distributions of univariate data (dotplots,back-to-back stemplots, parallel boxplots)

1. Comparing center and spread: within group,between group variation

2. Comparing clusters and gaps

3. Comparing outliers and other unusual features

See the Robert DE BEAUCHAMP Lord of Hache Entry for this Couple's Children and Descendants. Lucy DE MOHUN (Reynold DE Lord of Dunster 2, Reynold DE Lord of Dunster ((Sir)) 1) was born about 1240 in Dunster, Williton, Somerset, England. Lucy married (Sir) John DE GREY (See Link for Ancestry), son of Richard DE GREY Lord Of Codnor and Lucy DE. Descendants of mahunclan. Gilbert married Agnes MacDonald circa 1380. Daughter of John MacDonald and Grand daughter of Angus Og, Lord of the Isles who was the founder of the MacDonald Family known as the 'Macdonalds of Glencoe', the descendants of whom were the victims of the 'Massacre of Glencoe'. Research by G Lewis-Dean indicates Agnes was a descendant of King.

4. Comparing shapes

D. Exploring bivariate data

1. Analyzing patterns in scatterplots

2. Correlation and linearity

3. Least-squares regression line

4. Residual plots, outliers and influential points

5. Transformations to achieve linearity:logarithmic and power transformations

E. Exploring categorical data

1. Frequency tables and bar charts

2. Marginal and joint frequencies for two-waytables

3. Conditional relative frequencies and association

4. Comparing distributions using bar charts

II. Sampling and Experimentation: Planning andconducting a study (10%–15%) Data must be collected according to awell-developed plan if valid information on aconjecture is to beobtained. This plan includes clarifying the question and decidingupon amethod of data collection and analysis.

A. Overview of methods of data collection

1. Census

2. Sample survey

3. Experiment

4. Observational study

B. Planning and conducting surveys

1. Characteristics of a well-designed andwell-conducted survey

2. Populations, samples and random selection

3. Sources of bias in sampling and surveys

4. Sampling methods, including simple randomsampling, stratified random sampling and cluster sampling

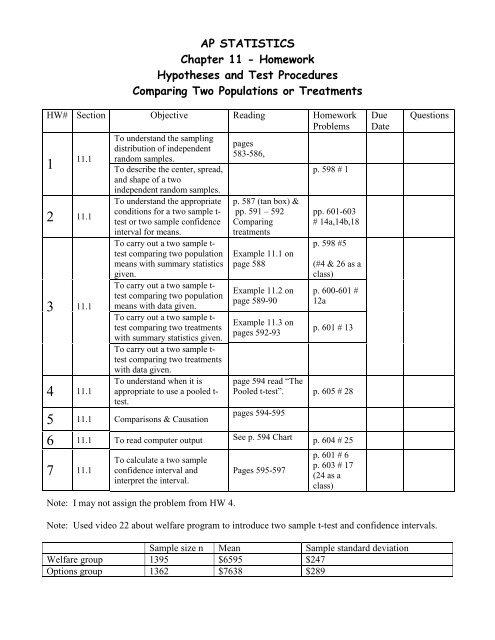
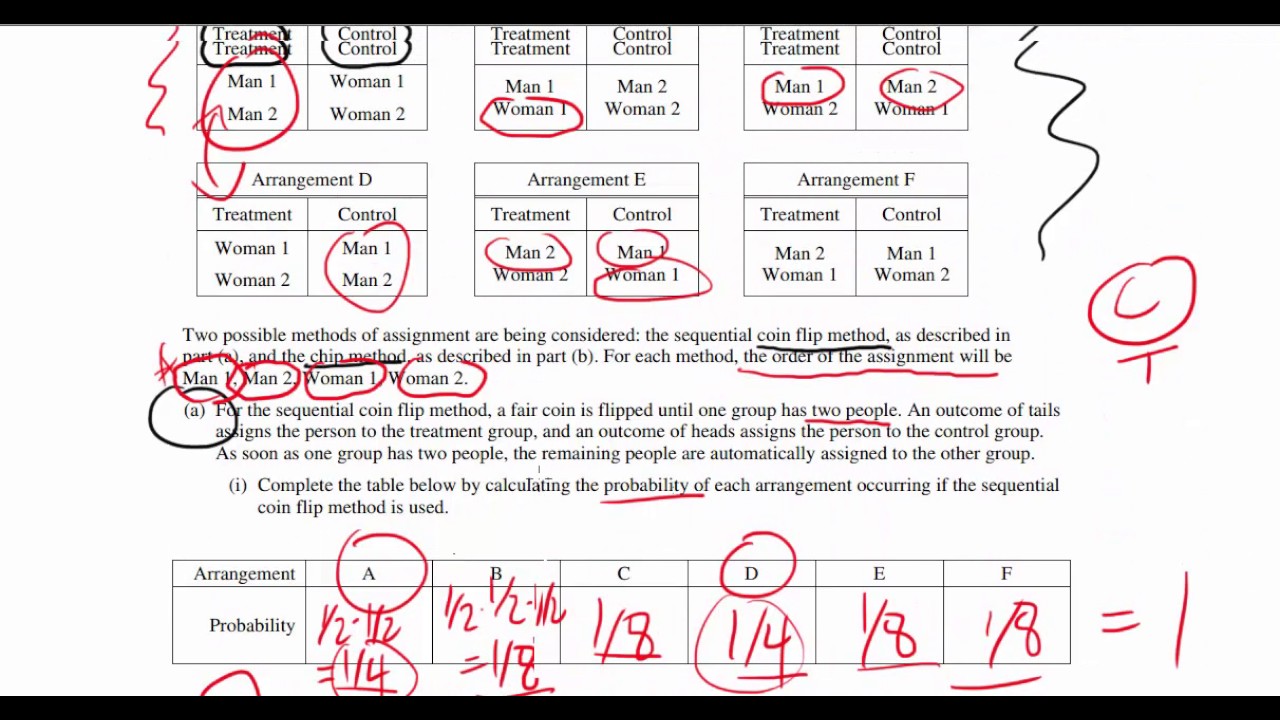
C. Planning and conducting experiments

1. Characteristics of a well-designed andwell-conducted experiment

2. Treatments, control groups, experimental units,random assignments and replication

3. Sources of bias and confounding, includingplacebo effect and blinding

4. Completely randomized design

5. Randomized block design, including matched pairsdesign

D. Generalizability ofresults and types of conclusions

III. Anticipating Patterns: Exploring random phenomenausing probability and simulation (20%–30%) Probability is the tool used foranticipating what the distribution of data shouldlook like under a givenmodel.

A. Probability

1. Interpreting probability, including long-runrelative frequency interpretation

2. “Law of Large Numbers” concept

3. Addition rule, multiplication rule, conditionalprobability and independence

4. Discrete random variables and their probabilitydistributions, including binomial and geometric

5. Simulation of random behavior and probabilitydistributions

6. Mean (expected value) and standard deviation ofa random variable, and linear transformation of a random variable

B. Combining independent random variables

1. Notion of independence versus dependence

2. Mean and standard deviation for sums anddifferences of independentrandom variables

C. The normal distribution

1. Properties of the normal distribution

2. Using tables of the normal distribution

3. The normal distribution as a model formeasurements

D. Sampling distributions

1. Sampling distribution of a sample proportion

2. Sampling distribution of a sample mean

3. Central Limit Theorem

4. Sampling distribution of a difference betweentwo independent sample proportions

5. Sampling distribution of a difference betweentwo independent ssamplemeans

6. Simulation of sampling distributions

7. t-distribution

8. Chi-square distribution

IV. Statistical Inference: Estimating populationparameters and testing hypotheses (30%–40%) Statistical inference guides theselection of appropriate models.

A. Estimation (point estimators and confidenceintervals)

1. Estimating population parameters and margins oferror

2. Properties of point estimators, includingunbiasedness and variability

3. Logic of confidence intervals, meaning of confidencelevel and confidence intervals, and properties of confidence intervals

4. Large sample confidence interval for aproportion

5. Large sampleconfidence interval for a difference between two proportions

6. Confidence interval for a mean

7. Confidence interval for a difference between twomeans (unpaired and paired)

8. Confidence interval for the slope of a least-squaresregression line

B. Tests of significance

1. Logic of significance testing, null and alternativehypotheses; p-values; one- and two-sided tests; concepts of Type I and Type IIerrors; concept of power

2. Large sample test for a proportion

3. Large sample test for a difference between twoproportions

4. Test for a mean

Ap Statistics

5. Test for a difference between two means(unpaired and paired)

6. Chi-square test for goodness of fit, homogeneityof proportions, and independence (one- and two-way tables)

Ap Statistics Formula Sheet

7. Test for the slope ofa least-squares regression line